Telescope Tube Length Galilean Equation. We are given that a Galilean telescope measures 9 cm from the objective to the eye-piece and the focal length of the objective is 15 cm. We have to find its. The above calculation assumes that the image is formed as a virtual image at infinity for comfortable viewing; this is the standard practice. The calculation involves application of.
The telescope tube length Galilean equation is a mathematical formula used to determine the required tube length of a Galilean telescope. A Galilean telescope is an optical device that uses two curved mirrors to magnify an object. The Galilean telescope is named after the Italian astronomer Galileo Galilei, who invented it in 1609.
Also Read
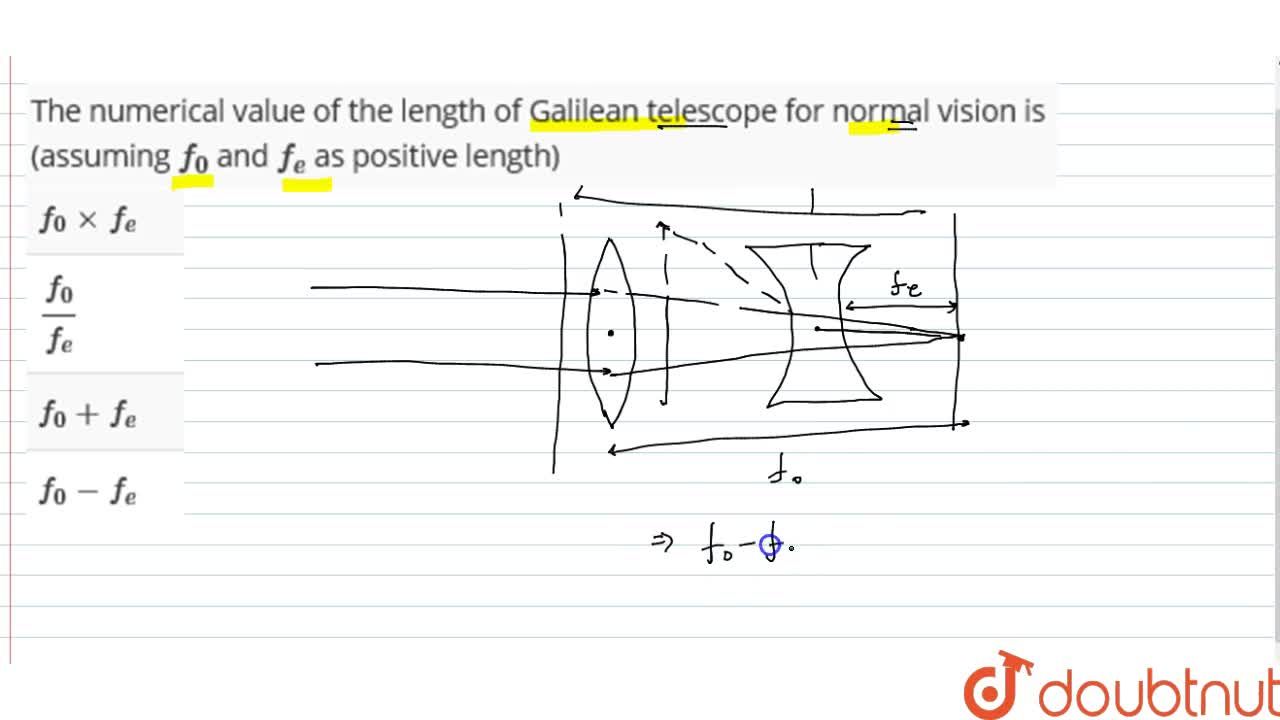
The equation for calculating the required tube length of a Galilean telescope is:
L = (f1 × f2) ÷ (f1 + f2)
Where:
- L is the tube length of the telescope, in millimeters.
- f1 is the focal length of the front mirror, in millimeters.
- f2 is the focal length of the rear mirror, in millimeters.
To calculate the required tube length of a Galilean telescope, you need to know the focal lengths of both the front and rear mirrors. The focal length of a mirror is the distance from the center of the mirror to the point where the light rays converge. Once you know the focal lengths of the two mirrors, you can plug them into the equation to calculate the required tube length.
For example, if you have a telescope with a front mirror with a focal length of 100 millimeters, and a rear mirror with a focal length of 50 millimeters, the required tube length of the telescope would be:
L = (100 × 50) ÷ (100 + 50) = 25 millimeters
Uses of the Telescope Tube Length Galilean Equation
The telescope tube length Galilean equation is used mainly in the design and construction of Galilean telescopes. By knowing the required tube length of a telescope, designers and engineers can more accurately plan and build a telescope that will produce the desired level of magnification. The equation can also be used to determine the magnification power of a telescope, by calculating the ratio of the focal length of the front mirror to the focal length of the rear mirror.
The telescope tube length Galilean equation is also used in the design and construction of other optical instruments, such as binoculars and microscopes. The equation can be used to determine the required tube length of these instruments, as well as their magnification power.
The telescope tube length Galilean equation is a useful tool for anyone involved in the design and construction of optical instruments. By understanding the equation and knowing the focal lengths of the mirrors, designers and engineers can accurately calculate the required tube length of a telescope, binoculars, or microscope, as well as its magnification power.
Question 22 Galilean Telescope Magnification
A Galilean telescope with a +5 D objective and a -20 D eyepiece produces an image with what magnification and direction? a) 4x, erect b) 4x, inverted c) 100x, erect d) 100x, inverted Answer a)
lens (see the lens equation and convince yourself of this) 1. To properly magnify, the eyepiece must be placed at a specific distance away from the focal point of the. Focal length is the distance the light travels to go from the entry to the exit point in a telescope. The focal length is important to determine the magnification and field. , Telescope Tube Length Galilean Equation.












